Lorentz Contraction
from Meta Research Bulletin, 2003 Vol. 12 Issue 3
Tom Van Flandern/Meta Research
<
Abstract. Lorentz contraction is not a change in the physical length of rods or meter sticks. Rather, it is an illusion introduced in special relativity by the lack of remote simultaneity. In Lorentzian relativity, elysium (the light-carrying medium) is entrained and time is universal, so the need for a Lorentz contraction vanishes.
Introduction
According to Einstein's special relativity (SR), the motion of a body relative to an observer produces time dilation – a slowing of the rate of passage of time, and length contraction – a compression along the direction of motion. For a moving extended body or an entire inertial frame in motion, SR also predicts a time desynchronization effect, sometimes referred to as a lack of remote simultaneity. The moving body or frame is unaware of any changes in itself or its own time, but instead sees the same kind of effects happening to the observer and all bodies in the original frame. The amount of these effects is given by the Lorentz transformation equations.
A variety of independent experiments, 11 in all, have tested and verified that the predicted "time dilation" effect occurs at least for atomic clocks, which do slow their rate of ticking with motion. [[1]] Strictly, it has not been verified that anything happens to time itself. Likewise, the reciprocity of the effect from moving frame back to laboratory frame and the time desynchronization effects have not been separately verified. As for length contraction, its existence is inferred, but it has also never been seen directly in any experiment.
We here consider the motivation for proposing length contraction and its physical nature. In the end, we will conclude that length contraction does not exist.
Time desynchronization
An important feature of SR is that time will appear desynchronized in any other inertial frame with a motion relative to the observer's own inertial frame. [[2]] Specifically, if the nearest clock in a moving frame with a train of clocks is synchronized with a clock at rest at the observer, then receding objects in the moving frame will be experiencing time in the observer's past, while approaching objects in the moving frame will be experiencing time in the observer's future. See Figure 1. The greater the distance, the greater is the time discrepancy. The fact that the finite speed of light prevents the observer from viewing into the past or future does not diminish the presumed reality of these time differences in SR.
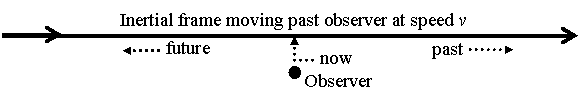
Figure 1. In SR, time will appear desynchronized with the present in any inertial frame with a motion relative to an observer.
Consider any object (say, a rod) in the passing frame, oriented along the direction of relative motion. Then according to SR, a snapshot of the rod taken by the stationary observer will not show the length of the rod all at one instant of "rod time", but will see one end at a different moment of moving-frame time than the other end. If the rod had stripes of different colors painted lengthwise from end-to-end and was rotating around its long axis, the observer would see the rod's colored stripes apparently twisted – not because the rod is twisted, but because different parts are seen at different rod-times during the rotation, all at a single observer instant.
Effect on a moving rod
As this pertains to Lorentz contraction, consider the consequences of this desynchronization of time in a relatively moving frame for the appearance of the moving rod as seen by the fixed observer. Suppose the rod's leading end is labeled A and its trailing end is labeled B. See Figure 2.

Figure 2. At any one instant, the observer will see a moving, rotating rod at progressively later times along its length from A to B. This makes the rod look shorter and its stripes appear twisted.
The rod's stripe is actually linear from one end to the other, with no wrapping. However, because of desynchronization, the observer will see end A as it was at some time in the moving frame (depending on location), and the observer will see end B as it was at some later time. So time along the rod appears different from place to place to the fixed observer. And that makes the stripe appear twisted as the rod rotates in time. What the observer sees of the moving rigid rod in an instant is the same as a video camera would see if it panned slowly along the length of a non-moving-but-rotating rod from end A to end B over some finite period of time.
A further consequence of this desynchronization is that end B will always be seen as it was at a later time moment than is seen for end A. But because B is moving in the same direction as A, a later moment will bring end B closer to where end A was a bit earlier. In other words, the rod will always appear contracted in length because the leading end is seen at an earlier time than the trailing end. This applies whether the rod is approaching or receding from the observer.

Pole and barn paradox
With this thought in mind, we can now readily understand the famous "pole and barn paradox" (Figure 3). Consider a 21-meter pole approaching a barn with doors open at both ends, 7 meters apart. Let the pole's speed be 99% of the speed of light so that the length contraction factor is 7. Then the observer in the barn's frame sees the length-contracted pole as only 3 meters long, and argues that both doors can be slammed shut while the pole is inside. But the pole regards itself as at rest and sees the barn approaching at 99% of the speed of light. The pole therefore thinks the barn is length-contracted to only 1 meter long between doors, and therefore cannot possibly contain the pole. Both views cannot be correct – hence, the paradox.
The resolution of the paradox comes from time desynchronization. The pole only appears contracted because the moment its leading tip reaches the far door of the barn is earlier than the moment its trailing tip passes by the near door. There is no single moment of time in either frame when the pole could actually be fully contained within the barn.
However, the clear implication of our considerations here is that length contraction is not a physical shortening, but is merely an observational consequence of time desynchronization. In SR, physical bodies do not actually change dimensions. This also explains why “length contraction” does not flatten spherical bodies in their direction of motion, making their rotation about a perpendicular axis irregular (an effect called “Thomas precession”). [[3]]
Length contraction in Lorentzian relativity
Our next question is: what does Lorentzian relativity (LR) say about length contraction? [[4]] LR agrees with all existing experimental evidence at least as well as SR, yet has no time desynchronization. As we see from the above considerations, the absence of time desynchronization means that LR has no length contraction either.
The original motivation for length contraction was in the interpretation of the Michelson-Morley experiment. In it, each half of a split light-beam travels along one of two equal-length, perpendicular arms of an interferometer and bounces off mirrors back to an observer. Surprisingly, both beams arrived back at the same time and produced no interference fringe shifts, even if the interferometer was turned to different orientations. Yet the Earth is moving at a speed of no less than 30 km/s in its orbit around the Sun, not to mention possible higher speeds in its Galactic orbit. So the round-trip travel time for a light beam through a light-carrying medium (aether) was expected to take longer when moving along the direction of observer motion than when moving perpendicular to that direction, for the same reason that a round-trip in a canoe takes longer when the stream is flowing than when the water is still. Yet observations show that the round-trip times for light beams in any direction were actually the same. This was explained in SR by length contraction along the direction of motion, making the interferometer arms shorter by just enough to compensate for the otherwise-longer travel time through the moving aether.
In LR, because there is no length contraction, it cannot be invoked to explain the lack of fringe shifts in the Michelson-Morley (M-M) experiment. But then the absence of fringe shifts implies that Earth has no motion relative to the aether. However, this is not a problem for LR. As is now becoming well known, LR recognizes the local gravitational potential field, also known as "elysium", as the light-carrying medium [[5], [6],[7]]. And that local field has no motion with respect to the Earth's center of mass. So the M-M experiment shows no fringe shifts because local elysium is entrained by the Earth and at rest with respect to it.
An exception is that Earth does rotate with respect to its own gravity field. However, rotation does produce fringe shifts in an M-M-type experiment. This is known as the Sagnac effect, which was first seen in 1913 in the laboratory using a rotating platform for the M-M experiment. The same effect was later replicated in the Michelson-Gale experiment in 1925 using the actual rotation of the Earth to rotate the M-M interferometer. So LR predicts the absence and presence of fringe shifts just as experiments confirm they should be.
Conclusions
We conclude that length contraction, which has never been seen per se in any experiment, is not a real, physical effect. In SR, it is merely a by-product of time desynchronization (the lack of remote simultaneity), and is therefore illusory. In LR, length contraction does not exist, but is not needed because elysium (the light-carrying medium) is the same as the local gravitational potential field, and is entrained by the Earth. So no fringe shifts are expected in a Michelson-Morley-type experiment unless there is rotation, which is just what experiments show.
Last updated 2007/08/25
References
[1] T. Van Flandern (1998), "What the Global Positioning System tells us about relativity", in Open Questions in Relativistic Physics, F. Selleri, ed., Apeiron, Montreal, 81-90; also at http://metaresearch.org/cosmology/cosmology2/what-the-global-positioning-system-tells-us-about-relativity
[2] T. Van Flandern (2003), "What the Global Positioning System tells us about the twins paradox", Apeiron 10, http://redshift.vif.com/JournalFiles/V10NO1PDF/V10N1TVF.pdf; also at http://metaresearch.org/cosmology/gravity2/what-the-global-positioning-system-tells-us-about-the-twins-paradox.
[3] Math Pages, “Thomas precession”, http://www.mathpages.com/rr/s2-11/2-11.htm.
[4] T. Van Flandern (2005), “Is faster-than-light propagation allowed by the laws of physics? (A primer on Lorentzian relativity)”, Infinite Energy 59:31-33; also at http://metaresearch.org/cosmology/gravity2/is-faster-than-light-propagation-allowed-by-the-laws-of-physics.
[5] T. Van Flandern (2004), "21st century gravity: a deeper understanding of why apples fall from trees”, J.Wash.Acad.Sci. 90:108-125; reprinted in J.NewEnergy 7:179-187.
[6] T. Van Flandern & J.P. Vigier (2002), “Experimental Repeal of the Speed Limit for Gravitational, Electrodynamic, and Quantum Field Interactions”, Found.Phys. 32:1031-1068.
[7] T. Van Flandern (2002), “Gravity”, in Pushing Gravity: new perspectives of Le Sage’s theory of gravitation, M.R. Edwards, ed., C. Roy Keys Inc., Montreal, 93-122.
