Does Gravity Have Inertia?

Tom Van Flandern / Meta Research

This email address is being protected from spambots. You need JavaScript enabled to view it.

Abstract. » Gravity makes heavy and light bodies fall at the same rate. Gravity obeys the “equivalence principle”, and is just "curved space-time geometry" in geometric general relativity. But space-time curvature alone cannot initiate motion, and changes in momentum still require a force acting. Moreover, gravity can deviate slightly from the “equivalence principle”, and “space-time” is really just proper time and does not involve any curvature of space. The Le Sage “pushing gravity” concept is a better way to explain the physics of gravity. For forces other than gravity, the momentum transferred must be shared by all particles in the target body, producing what we call “inertia” -- a simple dilution of momentum. Gravity obeys the “transparency principle”, allowing momentum to be transferred directly to each particle. Without need for dilution of momentum, gravity has no inertia. «

Gravity is different from the other known forces of nature. All bodies, big and small, accelerate at equal rates in any given gravitational field. That property is opposite to our everyday experience, in which more massive bodies require more work to move or accelerate than less massive ones. That gravity accelerates masses of all size with equal ease is so anti-intuitive that people universally believed otherwise until Galileo’s demonstration at the Leaning Tower of Pisa. He simultaneously dropped a heavy and a light mass (both heavy enough that air resistance was not a factor), and observers below tried to time which hit first and by how much. But to the astonishment of the observers, who were certain that the heavier body would fall faster, the two masses reached the ground at the same time.

David Scott, an Apollo 15 (1971) astronaut, did a unique, modern-day version of this same basic experiment by simultaneously dropping a hammer and an eagle feather while standing on the Moon. Because the Moon has no atmosphere, the fall of the feather is due to gravity only, not slowed by air resistance. And because the Moon has only 1/6 of Earth’s surface gravity, the falls of both objects were slowed by a factor of six. But again, the heavy and light objects hit the ground at the same time.

When the nature of gravitation was being considered around the beginning of the 20th century, the uniqueness of this property played a major role in that thinking. Einstein formulated the principle of equivalence – that a uniform acceleration and a gravitational field were indistinguishable to an enclosed observer. No experiment from inside a closed box could tell, he reasoned, whether the box was resting on the surface of a massive body or was being accelerated by rockets through outer space. Either way, a downward, gravity-like force would be felt. Likewise, someone in a freely falling elevator would accelerate downward but feel no force, and might conclude that he was floating in outer space without any acceleration.

Einstein used this equivalence principle to conclude that gravity is not a force like the other forces of nature, but is instead a curvature of space-time near large source masses. Because all parts of a target body simultaneously experience this curvature as they move, the change in the target body’s motion would be the same no matter how big or small that body is. This was a new and unique way of thinking about gravity.

The problems with this curved space-time view are several. But the most basic of them all is that a body at rest in a gravitational field has no cause to commence motion because curvature does not induce motion unless a force acts. For example, if “curved space-time” were visualized as a rubber sheet with a dent, then a body at rest on the side of the dent would remain stuck there unless a force (such as gravity underneath the rubber sheet) acted to make it move. In open space with no gravity acting, the body would have no sense of which direction was “down” and no reason to move. [[1]]

A second problem with curved space-time (or curved anything) causing motion by itself is that motion is momentum, and momentum cannot be created from nothing. So the curved space-time (or curved whatever) must still apply a force; i.e., it must have momentum of its own in the form of moving parts. Creating momentum or anything from nothing requires a miracle, and postulating that the curved space-time applies a force or has moving parts defeats the value of this “pure geometry” mechanism as an explanation for gravity. With a force applied, we would be back to wondering why big masses and small all fell (or curved) by the same amount, unlike the action of other forces of nature. [[1]]

A third problem with curved space-time is that the acceleration of a body in a gravitational field is not completely independent of the body’s own mass. (This point is unrelated to the back-acceleration any small body produces on the source mass, which may affect the relative acceleration between two bodies, but not the acceleration of the target body relative to an inertial frame such as that provided by the distant stars.) Equations of motion in general relativity (GR) [[2]] show that a body’s mass does (at order ) very slightly affect its own acceleration, violating the equivalence principle. Experimentally, this violation of the equivalence of acceleration and gravitational fields has been observed with neutron interferometers. [[3]] So in addition to the difficulty this idea poses for consistency with physical principles, it is theoretically and experimentally incorrect too.

Some relativists may argue that “space-time” is not simply space plus time, but a higher-level concept that includes the notion of “time”, so the physical principles do not apply. However, the physical principles arise from logic alone and should be immutable, in contrast to the laws of physics, which can change as knowledge improves. [[4]] Moreover, “space-time” is a mathematical concept, which amounts to a fancy way of referring to proper time in relativity (the time kept by perfect clocks), and does not involve any curvature of space. To show this, consider the following mathematical and physical arguments.

Let dT be a coordinate time interval (an idealized time in some specific reference frame) for a moving body, and let (dX, dY, dZ) be the change in the body’s space coordinates during that time interval. Next, let ds be a path length in “space-time” for the body during the same interval; and let c be the speed of light. Then the standard relation between space, time, and “space-time” (with no gravity acting) is:

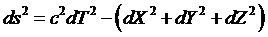[1]

Multiplying the coordinate time interval by the speed of light has turned time into a length-like coordinate (meaning a time interval measured in meters, not to be confused with a "space-like interval" as used in GR). This change of units allows the time interval to be combined with the coordinates for the three spatial dimensions. However, the presence of a minus sign makes the combination un-length-like; i.e., not the equivalent of space plus time treated as comparable length-like coordinates. So to see the physical meaning of the space-time parameter s, first note that the parentheses enclose the square of the distance traveled by the body. But distance is just velocity v times the time interval dT: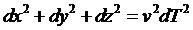 . So [1] becomes:

Moreover, if the body travels through a gravitational field having potential f (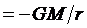; note the minus sign, following the astronomical convention wherein potentials are negative) at a distance r from a source mass M, then s is a “curved” space-time path length along a geodesic path (a free-fall path through a gravitational field), and our preceding formula generalizes to what is called the “Schwarzschild metric”:

[2]

Finally, divide each term by c2, which converts the length-like interval ds into a time-like interval that we can readily identify as the elapsed proper time for the body, dt, as defined in the theory of relativity:

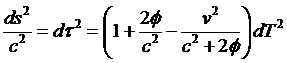[3]

In this form, we can see the space-time interval ds as a purely time-like interval dt that was merely made to look length-like through multiplying it by c. This is what we mean by saying that curved space-time does not involve a curvature of space. The only effects in the relation between coordinate time and “space-time” are the clock-slowing effects of velocity and gravitational potential.

Equations [2], [3] can also be used in a different way by setting the elapsed coordinate time dT = 0 to give what GR calls a “space-like interval”. But we must first cancel the explicit dT in [3] with the implicit dT in the denominator of , where du is the Euclidean distance traveled in time dT. We can simplify what is left by introducing the contraction parameter 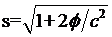. Then [3] reduces to: , where t is proper time. This is an expression for the velocity of a lightwave through a potential field, and shows that lightwaves slow when passing through a stronger gravitational potential. It still does not involve space curvature.

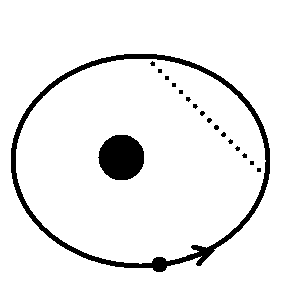
Because the point about spacetime not including space is of some importance, we will illustrate it physically as well. Consider the geodesic (orbital) path of the Earth with respect to the Sun in Figure 1. If we choose any two points along that path (call them A and B), note that a straight line between A and B (as could be represented by a taut rope) is a shorter path through space than the geodesic path. Precisely the same remarks would be true if the Earth were replaced by a photon whose path is bent with respect to space as it passes the Sun – a taut rope takes a shorter path through space than the photon does. The extra bending is most easily explained as a refraction effect in the space-time or light-carrying medium. [ [5], [6]] This again illustrates that “curved space-time” geodesic paths do not involve any curvature of space.

The contrary viewpoint in many textbooks has been a source of confusion for physics students for the last generation. For an extreme expression of this contrary viewpoint in support of the geometric interpretation of GR, see opinions by Robert Wald. [[7]] Yet the fact that space does not curve just because "space-time" does would come as a surprise to many students of the history of relativity, who tend to think as Riemann did. However, even in Misner, Thorne & Wheeler's text that popularized the geometric interpretation of GR, [ [8]] we find the following caution: "But if there was one reason more than any other why [Riemann] failed to make the decisive connection between gravitation and curvature, it was this, that he thought of space and the curvature of space, not of spacetime and the curvature of spacetime."

This is an important concept. If the curved path of a body through space is not caused by a curvature of space, then space remains Euclidean (flat) and an external force is still required to produce and explain any deviation from straight line motion. Moreover, some explanation other than curved space is needed to understand the equivalence-principle-like property of gravity.

Fortunately, another explanation of the equivalence principle and of gravitation itself, consistent with general relativity, is available. It is based on the Le Sage model, in which space is filled with a flux of extremely tiny, extremely fast particles called “gravitons”. [[9]] The apple falls from the tree because it is struck by more gravitons from above than from below because Earth blocks some gravitons from getting through from below. And any two bodies in space shadow one another from some graviton impacts, resulting in a net push toward one another. The special GR effects (light-bending, gravitational redshift, radar time delay, pericenter advance) are provided by an optical, light-carrying medium called “elysium” through the phenomenon of refraction because gravity makes the medium denser near masses.

To understand why gravity appears to obey an equivalence principle, we first need to understand why other forces of nature do not. Visualize what happens to a body composed of innumerable atoms when we push it. Obviously, the push makes direct contact only with a relatively small number of atoms. Those contacted atoms are set in motion by the push. But before they travel very far, they collide with other atoms and pass along some of their momentum. Those atoms in turn collide with other atoms, and so on, until all atoms comprising the body are set into motion. This transfer of momentum from atom to atom occurs so rapidly that it appears to be instantaneous to our senses. But of course, the pressure wave resulting from the original push travels through the body at the speed of sound for that body, always less than the speed of light; and the far side of the body does not begin to move until the pressure wave arrives there. For example, the speed of sound in iron or soft steel is about 5000 m/s.

So whatever force is applied to the original points of contact, this force transfers momentum that must ultimately be shared equally by all the atoms of the body. The more atoms present, the more sharing, with correspondingly less momentum for each atom. Because the mass of the body is the sum of the masses of all its atoms, we can now see in an intuitive way why the resistance to new motion (acceleration) of the body is inversely proportional to its own mass. The more mass, the greater the division of any momentum applied to the body among its atoms, leaving less momentum for each atom.

The essence of “inertia” is the resistance of a body to change from a state of rest or steady linear motion. The dilution of momentum just described is why bodies appear to have inertia in proportion to their own masses. In contrast to Mach’s famous conjecture that inertia originates in the distant mass of the universe, we see here that inertia is produced entirely within the affected body and is caused by the dilution of momentum among more constituents of a body than are directly affected by the applied force.

Gravity has no such dilution. The obvious explanation for this characteristic is that Le Sage-type momentum carriers of gravitational force are so small that they easily reach every part of the interior of the affected body, yet move so fast that they still carry appreciable momentum despite their small size. We call this the “transparency principle”, wherein every constituent of a body is equally accessible to a force. Although gravitons are theoretical, the concept of transparency is not. Neutrinos are an example of entities that usually fly easily through planet-sized masses without noticing, but occasionally are absorbed.

When the transparency principle operates, a force is applied equally to every constituent of the body. There is therefore no need for constituents to carry a pressure wave to their neighbors because all constituents are affected equally. Under those circumstances, it does not matter how many constituents are present. There is no dilution of momentum, so gravitational acceleration is the same for bodies of any mass.

And that is a sufficient reason for gravitation to operate as if the equivalence principle were in effect. Bodies of all masses fall at the same rates from the Tower of Pisa because the acceleration applied by Earth’s gravity is the same for each constituent, and does not depend on the number of constituents or on the body’s mass. Inertia (the amount of resistance to a change of motion for a target body) is a characteristic of the particular force being applied, and not something intrinsic to the body that would affect its response to all external forces.

We therefore answer our title question in the negative. In gravitation, any momentum transferred to a body by an external force suffers no dilution and is applied undiminished to each body constituent. Each such momentum transfer is an impulse. A continuum of impulses produces acceleration. And gravitational acceleration is independent of the mass of the affected body. So gravitation operates without inertia.

[1] T. Van Flandern & J.P. Vigier (2002), “Experimental Repeal of the Speed Limit for Gravitational, Electrodynamic, and Quantum Field Interactions”, Found.Phys. 32(#7): 1031-1068.

[2] C.W. Misner, K.S. Thorne & J.A. Wheeler (1973), Gravitation, W.H. Freeman & Co., San Francisco, 1095.

[3] D.M. Greenberger & A.W. Overhauser (1980), “The role of gravity in quantum theory”, Sci.Amer. 242 (May): 66.

[4] T. Van Flandern (2001), “Physics has its principles”, in Gravitation, Electromagnetism and Cosmology, K. Rudnicki, ed., C. Roy Keys Inc., Montreal, 87-101; also (2000), MetaRes.Bull. 9: 1-9.

[5] Sir A. Eddington (1920), Space, Time and Gravitation, Cambridge Univ. Press (reprinted 1987), Cambridge, 109.

[6] T. Van Flandern (2002), “Gravity”, in Pushing Gravity: New Perspectives on Le Sage’s Theory of Gravitation, Apeiron, Montreal, 93-122.

[7] R.M. Wald (1984), General Relativity, U. of Chicago Press, Chicago, 67.

[8] C.W. Misner, K.S. Thorne & J.A. Wheeler (1973), op.cit., 32-33.

[9] M. Edwards, ed. (2002), Pushing Gravity: New Perspectives on Le Sage's Theory of Gravitation, Apeiron Press, Montreal, 93-122.